Radar Installation
| Time Limit: 1000MS | Memory Limit: 10000K | |
| Total Submissions: 59563 | Accepted: 13430 |
Description
Assume the coasting is an infinite straight line. Land is in one side of coasting, sea in the other. Each small island is a point locating in the sea side. And any radar installation, locating on the coasting, can only cover d distance, so an island in the sea can be covered by a radius installation, if the distance between them is at most d.
We use Cartesian coordinate system, defining the coasting is the x-axis. The sea side is above x-axis, and the land side below. Given the position of each island in the sea, and given the distance of the coverage of the radar installation, your task is to write a program to find the minimal number of radar installations to cover all the islands. Note that the position of an island is represented by its x-y coordinates.
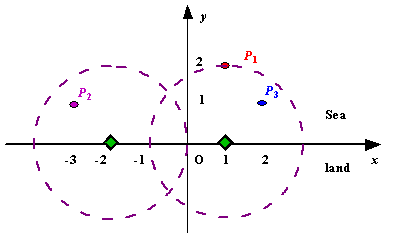
Figure A Sample Input of Radar Installations
We use Cartesian coordinate system, defining the coasting is the x-axis. The sea side is above x-axis, and the land side below. Given the position of each island in the sea, and given the distance of the coverage of the radar installation, your task is to write a program to find the minimal number of radar installations to cover all the islands. Note that the position of an island is represented by its x-y coordinates.

Figure A Sample Input of Radar Installations
Input
The input consists of several test cases. The first line of each case contains two integers n (1<=n<=1000) and d, where n is the number of islands in the sea and d is the distance of coverage of the radar installation. This is followed by n lines each containing two integers representing the coordinate of the position of each island. Then a blank line follows to separate the cases.
The input is terminated by a line containing pair of zeros
The input is terminated by a line containing pair of zeros
Output
For each test case output one line consisting of the test case number followed by the minimal number of radar installations needed. "-1" installation means no solution for that case.
Sample Input
3 2 1 2 -3 1 2 1 1 2 0 2 0 0
Sample Output
Case 1: 2 Case 2: 1
区间选点问题
#include <iostream> #include <algorithm> #include <cmath> #include <cstdio> using namespace std; #define N 1010 struct P { double x1,x2; bool operator < (const P &t)const { if(x2!=t.x2) return x2<t.x2; return x1>t.x1; } }p[N]; int main() { int n,r; int iCase=1; int end_flag; while(scanf("%d%d",&n,&r),n||r) { end_flag=0; for(int i=1;i<=n;i++) { double x,y; scanf("%lf%lf",&x,&y); if(y>r) end_flag=1; p[i].x1=x-sqrt(r*r-y*y); p[i].x2=x+sqrt(r*r-y*y); } printf("Case %d: ",iCase++); if(end_flag) { printf("-1 "); continue; } sort(p+1,p+n+1); int cnt=1; double tmp=p[1].x2; for(int i=2;i<=n;i++) { if(p[i].x1>tmp) { cnt++; tmp=p[i].x2; } } printf("%d ",cnt); } return 0; }