UVA211-The Domino Effect(dfs)

Problem UVA211-The Domino Effect

Accept：536  Submit：2504

Time Limit: 3000 mSec

 Problem Description

 

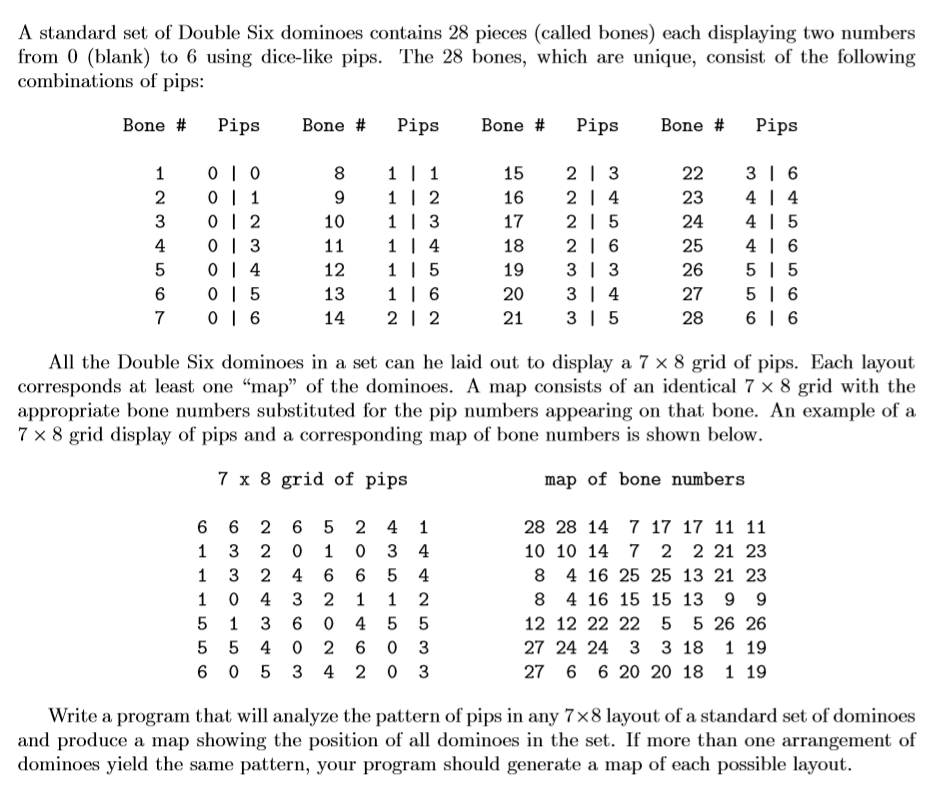
 Input

The input file will contain several of problem sets. Each set consists of seven lines of eight integers from 0 through 6, representing an observed pattern of pips. Each set is corresponds to a legitimate configuration of bones (there will be at least one map possible for each problem set). There is no intervening data separating the problem sets.

 Output

Correct output consists of a problem set label (beginning with Set #1) followed by an echo printing of the problem set itself. This is followed by a map label for the set and the map(s) which correspond to the problem set. (Multiple maps can be output in any order.) After all maps for a problem set have been printed, a summary line stating the number of possible maps appears. At least three lines are skipped between the output from different problem sets while at least one line separates the labels, echo printing, and maps within the same problem set.
Note: A sample input file of two problem sets along with the correct output are shown.

 Sample Input

5 4 3 6 5 3 4 6
0 6 0 1 2 3 1 1
3 2 6 5 0 4 2 0
5 3 6 2 3 2 0 6
4 0 4 1 0 0 4 1
5 2 2 4 4 1 6 5
5 5 3 6 1 2 3 1
4 2 5 2 6 3 5 4
5 0 4 3 1 4 1 1
1 2 3 0 2 2 2 2
1 4 0 1 3 5 6 5
4 0 6 0 3 6 6 5
4 0 1 6 4 0 3 0
6 5 3 6 2 1 5 3

 

 

 Sample Ouput

Layout #1:

   5   4   3   6   5   3   4   6
   0   6   0   1   2   3   1   1
   3   2   6   5   0   4   2   0
   5   3   6   2   3   2   0   6
   4   0   4   1   0   0   4   1
   5   2   2   4   4   1   6   5
   5   5   3   6   1   2   3   1

 

Maps resulting from layout #1 are:

 

   6  20  20  27  27  19  25  25
   6  18   2   2   3  19   8   8
  21  18  28  17   3  16  16   7
  21   4  28  17  15  15   5   7
  24   4  11  11   1   1   5  12
  24  14  14  23  23  13  13  12
  26  26  22  22   9   9  10  10

 

There are 1 solution(s) for layout #1.

 

 

 

Layout #2:

   4   2   5   2   6   3   5   4
   5   0   4   3   1   4   1   1
   1   2   3   0   2   2   2   2
   1   4   0   1   3   5   6   5
   4   0   6   0   3   6   6   5
   4   0   1   6   4   0   3   0
   6   5   3   6   2   1   5   3

 

Maps resulting from layout #2 are:

 

  16  16  24  18  18  20  12  11
   6   6  24  10  10  20  12  11
   8  15  15   3   3  17  14  14
   8   5   5   2  19  17  28  26
  23   1  13   2  19   7  28  26
  23   1  13  25  25   7   4   4
  27  27  22  22   9   9  21  21

 

  16  16  24  18  18  20  12  11
   6   6  24  10  10  20  12  11
   8  15  15   3   3  17  14  14
   8   5   5   2  19  17  28  26
  23   1  13   2  19   7  28  26
  23   1  13  25  25   7  21   4
  27  27  22  22   9   9  21   4

 

There are 2 solution(s) for layout #2.

 

题解：非常暴力的搜索。想了半天剪枝怎么剪，最后发现不用剪......（想想也对，可能的情况确实比较少）

 

#include <bits/stdc++.h>

using namespace std;

const int maxn = 10;
const int n = 7,m = 8;

int table[maxn][maxn];
int gra[maxn][maxn],ans[maxn][maxn];
int _count;
bool vis[maxn][maxn];
bool used[maxn<<2];
int dx[] = {0,1};
int dy[] = {1,0};

void init(){
    memset(table,0,sizeof(table));
    memset(vis,false,sizeof(vis));
    memset(used,false,sizeof(used));
    int i = 0,j = 0,cnt = 1;
    for(int len = 7;len >= 0;len--){
        for(int p = j;p < 7;p++){
            table[i][p] = table[p][i] = cnt++;
        }
        i++,j++;
    }
}

void dfs(int x,int y,int cnt){
    if(cnt == 28){
        _count++;
        for(int i = 0;i < n;i++){
            for(int j = 0;j < m;j++){
                printf("%4d",ans[i][j]);
            }
            printf("
");
        }
        printf("
");
        return;
    }

    if(y == m) x++,y = 0;
    if(vis[x][y]) dfs(x,y+1,cnt);
    else{
        for(int i = 0;i < 2;i++){
            int xx = x+dx[i],yy = y+dy[i];
            if(xx>=n || yy>=m) continue;
            if(vis[xx][yy] || used[table[gra[x][y]][gra[xx][yy]]]) continue;

            ans[x][y] = ans[xx][yy] = table[gra[x][y]][gra[xx][yy]];
            vis[x][y] = vis[xx][yy] = used[table[gra[x][y]][gra[xx][yy]]] = true;
            dfs(x,y+1,cnt+1);
            vis[x][y] = vis[xx][yy] = used[table[gra[x][y]][gra[xx][yy]]] = false;
        }
    }
}

int main()
{
#ifdef GEH
    freopen("input.txt","r",stdin);
#endif
    init();
    int iCase = 0;
    while(~scanf("%d",&gra[0][0])){
        for(int i = 0;i < n;i++){
            for(int j = 0;j < m;j++){
                if(i==0 && j==0) continue;
                scanf("%d",&gra[i][j]);
            }
        }

        if(iCase) printf("


");
        printf("Layout #%d:

",++iCase);
        for(int i = 0;i < n;i++){
            for(int j = 0;j < m;j++){
                printf("%4d",gra[i][j]);
            }
            printf("
");
        }
        printf("
");
        printf("Maps resulting from layout #%d are:

",iCase);
        _count = 0;
        dfs(0,0,0);
        printf("There are %d solution(s) for layout #%d.
",_count,iCase);
    }
    return 0;
}